*sigh*
Okay. What aerohead is arguing is that if we have a curve, a surface that is not the same shape as the curve but intersects it at 2 points will have similar or the same flow over it (hence the list of cars that supposedly intersect his template in profile view). Graphically:

Let's assume this curve has attached flow over it, and bisect it at two arbitrary points upstream and downstream of its average inclination and assume the car body ends at the line to the right:

Replace this curve with a flat surface intersecting it at the two red lines:
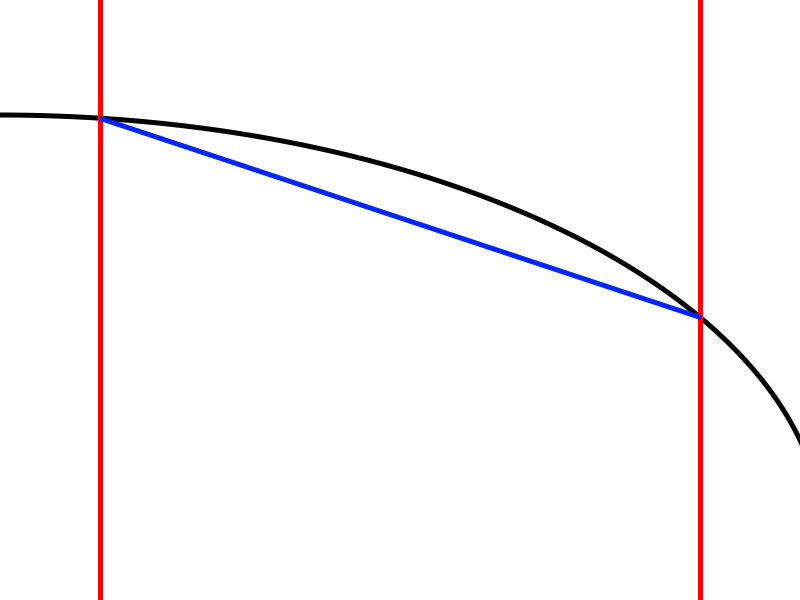
Or replace it with a flat surface that has a steeper angle of inclination and a spoiler, extending or standing up so that it still intersects the curve:

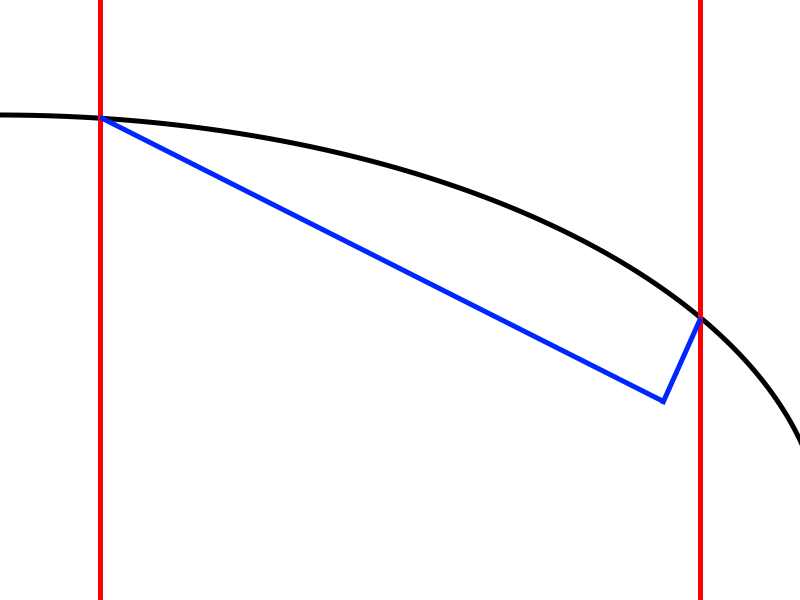

The argument is that the "outer" airflow will still follow the shape of the curve, not the real surface, that flow will recirculate on the real surface, and that the pressure on the real surface will be lower in front of the spoiler, higher in the "outer flow," and higher behind the spoiler, on the base.
First problem: this isn't how spoilers work. You can see this on your own car if you tape spoilers of various heights and shapes to it along with tufts on the rear window, and measure pressures on the window and base:

Here, a spoiler does
not cause the flow to recirculate on the window (or even just ahead of the spoiler) and outer flow to "skip" over the surface in a more-perfect "template" shape. The car has attached flow over the window with or without this spoiler, despite its having a faster curve than the "template" (which tells us something about the "template's" ability to predict attached and separated flows, another of its claimed uses on this site...). With the spoiler in place, pressure increases on the window (and roof) ahead of it.
Further, take this argument to its logical conclusion: if the airflow will follow a "perfect" shape regardless of the actual body shape between these two points, then these:


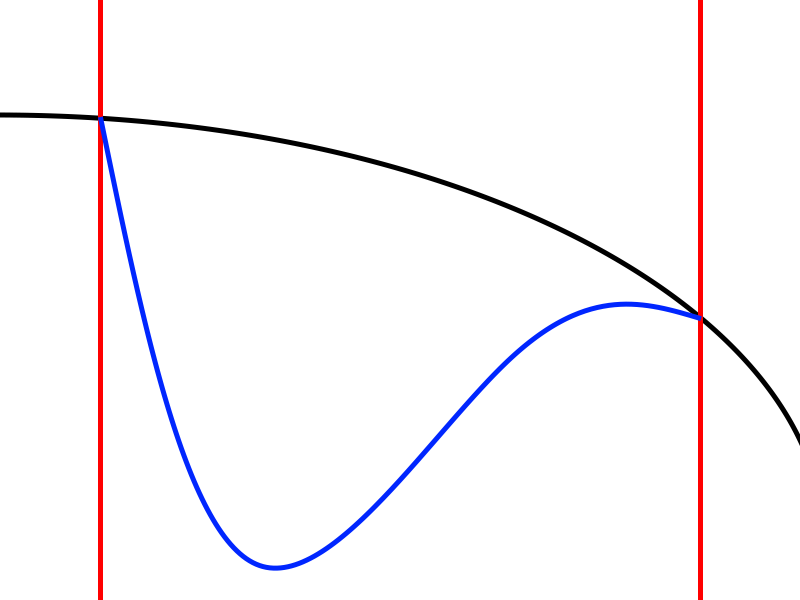
...would be aerodynamically identical to the actual curve. This is simply not true. Aerodynamic parameters such as pressure and velocity are
path-dependent. In other words, it matters what happens
between two points, not just
at the points.