Quote:
Originally Posted by trebuchet03

What's the Reynolds number for a dimpled golf ball, swimmer in water, cyclist?
What's the Reynolds number for a car?
If they're close - then these features may directly apply for the same reasons. The last paragraph is most telling  |
I stayed up until 1am last night doing research and I think the only benefit I can see from dimpling would be from testing.
I would suspect the rear section could gain the most and maybe the trailing edge of the mirrors, sides of the front bumper and a body pan could benefit from a lower drag coefficient, especially those areas which have a greater curve.
To test Reynolds we need to use the following formula:
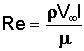
Where:
r = atmospheric density
V¥ = velocity
l = reference length (in the case of a sphere, this variable is defined as the diameter)
m = viscosity (or friction) (the funny symbol for those not familiar with it)
I found another
page which explains what golf ball dimpling does. Essentially the dimpling creates a turbulent air flow over the surface of the ball (like applying a layer of oil to a ball bearing) this creates a similar effect to an airplane wing which decreases trailing wind disturbance. If this holds true, what are the effects of 1/4 of a golf ball shaped area (the right and left front bumper area in some cars). This is clearly raising more questions than answers so the research continues.