My personal thoughts turn to modelability. How accurately can you define the surface? The solution seems to be a octahedral-based geodesic structure.
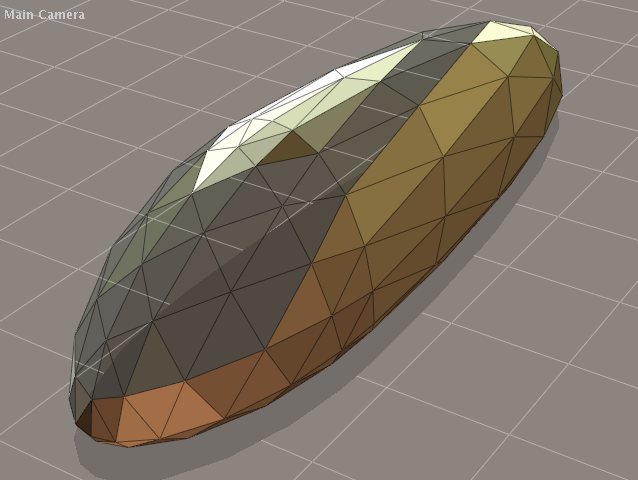
If you like I can show* the progression from a sphere, to a 30/70 front-rear split, cross-section that is prolated above the equator and oblated below and flat-bottomed, with an overall resemblance to a humped-backed Dymaxion.
This relies on a linear transformation fore and aft of 'center'. With added complexity you could have a non-linear transform produce a reflex curve and stinger tail. The handful of data points that define
http://ecomodder.com/forum/showthrea...tml#post373749 the [un-mirrored] half shape can still be calculated to as many decimal point as you require.
Further transformation. I haven't found a way to apply this to the octahedral geodesic, but it could probably be done station-by-station. A squircle can be anywhere between a square and a circle.

This can resolve packaging issues, like windshield headers without upper corners, and etc. The subject is covered here:
http://ecomodder.com/forum/showthrea...ion-23333.html
edit:
*
Permalink #29